Relative Velocity

A boat which has a speed of $5 \mathrm{km} {\mathrm{h}}^{-1}$ in still water crosses a river of width $1 \mathrm{km}$ along the shortest possible path in $15$ minutes. The velocity of the river water (in $\mathrm{km} {\mathrm{h}}^{-1}$) is

A person swims in a river aiming to reach exactly opposite point on the bank of a river. His speed of swimming is $0.5\hspace{0.17em}\mathrm{m}\mathrm{\hspace{0.17em}}{\mathrm{s}}^{-1}$ at an angle $120°$ with the direction of flow of water. The speed of water in stream is:

A boat is sent across a river with a velocity of $8 \mathrm{km }{\mathrm{hr}}^{-1}$. If the resultant velocity of the boat is $10 \mathrm{km} {\mathrm{hr}}^{-1}$, then the velocity of the river is

A bus is moving with a speed of  $10m{s}^{-1}$  on a straight road . A scooterist wishes to overtake the bus in 100 s. If the bus is at a distance of 1 km from the scooterist, with what speed should the scooterist chase the bus?

A train of 150 meters long is going towards north direction at a speed of $10\frac{m}{s}$ . A parrot flies at the speed of $5\frac{m}{s}$ towards south direction parallel to the railway track. The time taken by the parrot to cross the train is

On a frictionless horizontal surface, assumed to be the $x – y$ plane, a small trolley $A$ is moving along a straight line parallel to the $y$– axis (see figure) with a constant velocity of  $\left(\sqrt{3}-1\right)m{s}^{-1}$ . At a particular instant, when the line $OA$ makes an angle of  $45°$  with the $x$-axis, a ball is thrown along the surface from the origin $O$. Its velocity makes an angle $\varphi$ with the $x$-axis and it hits the trolley.​
The motion of the ball is observed from the frame of the trolley and the velocity vector of the ball makes an angle $\varphi$ with the x-axis in this frame.


Find the speed of the ball with respect to the surface if  $\varphi =\frac{4\theta }{3}.$

On a frictionless horizontal surface, assumed to be the x-y plane, a small trolley A is moving along a straight line parallel to the y-axis (see figure) with a constant velocity of $\left(\sqrt{3}-1\right)m{s}^{-1}$. At a particular instant, when the line OA makes an angle of $45°$ with the x-axis, a ball is thrown along the surface from the origin O. Its velocity makes an angle $\varphi$ with the x-axis and it hits the trolley. The motion of the ball is observed from the frame of trolley. Calculate the angle $\theta$ made by the velocity vector of the ball with the x-axis in this frame.

A ball is thrown from a point with a speed $v_0$  at an angle of projection $\theta$ . from the same point and at the same instant, a person starts running with a constant speed  $\frac{v_0}{2}$ to catch the ball. will the person be able to catch the ball? If yes, what should be the angle of projection?

A river is flowing from west to east at a speed of $5 \mathrm{m}$ per minute. A man on the south bank of the river, capable of swimming at $10 \mathrm{m}$ per minute in still water, wants to swim across the river in the shortest time. He should swim in a direction

Four persons $K$, $L$, $M$, $N$ are initially at the four corners of a square of side $d$. Each person now moves with a uniform speed  $v$ in such a way that $K$ always moves directly towards $L$, L directly towards $M$, $M$ directly towards $N$, and $N$ directly towards $K$. The four persons will meet at a time

 An aeroplane is flying with the velocity of $V_1=800 {\mathrm{kmh}}^{-1}$ relative to the air towards south. A wind with velocity of $V_2=15 \mathrm{m} {\mathrm{s}}^{-1}$ is blowing from west to east. What is the velocity of the aeroplane with respect to the earth?
 

Three particles $A, B$ and $C$ are situated at the vertices of an equilateral triangle $ABC$ of side $d$ at $t=0 .$ Each of the particles moves with constant speed $v .$ $A$ always has its velocity along $A B, B$ along $BC$ and $C$ along $CA$. At what time will the particles meet each other?

A swimmer jumps from a bridge over a canal and swims $1 \mathrm{km}$ upstream. After that first $\mathrm{km}$, he passes a floating cork. He continues swimming for half an hour and then turns around and swims back to the bridge. The swimmer and the cork reach the bridge at the same time. The swimmer has been swimming at a constant speed. How fast does the water in the canal flow?

Two point masses A and B are moving in the same straight line. A moves with a uniform velocity $11 \mathrm{m} {\mathrm{s}}^{-1}$. B starts from rest at the instant it is $52.5 \mathrm{m}$ ahead of A and moves with a uniform acceleration of $1 \mathrm{m} {\mathrm{s}}^{-2}$. When will A catch B.
 

An airplane moves in a northwesterly direction at $125 \mathrm{mi}/\mathrm{hr}$ relative to the ground, due to the fact there is a westerly wind of $50 \mathrm{mi}/\mathrm{hr}$ relative to the ground. How fast and in what direction would the plane have traveled if there were no wind?

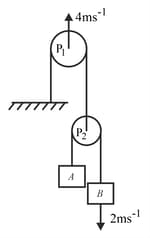
In the figure given below, calculate the velocity of block $A$ (in $\mathrm{m} {\mathrm{s}}^{-1}$). If the pulley $P_1$ has velocity $4 \mathrm{m} {\mathrm{s}}^{-1}$ upward and block $B$ has velocity $2 \mathrm{m} {\mathrm{s}}^{-1}$ downward.

​​​​​​​

Velocity of a boat with respect to a flowing river is $2\sqrt{3} \mathrm{km} {\mathrm{h}}^{-1}$ at an angle of $60°$ measured with the line perpendicular to the river flow direction. If the drift of the boat in reaching opposite bank of river with width $12 \mathrm{m}$ is zero, then velocity of river flow is

A lift whose cage is $3 \mathrm{m}$ high is moving up with an acceleration of $2 \mathrm{m} {\mathrm{s}}^{-2}$. A piece of stone is dropped from the top of the cage of the lift when its velocity is $8 \mathrm{m} {\mathrm{s}}^{-1}$. If $g=10 \mathrm{m} {\mathrm{s}}^{-2}$, then the stone will reach the floor of the lift after

A passenger in an open car travelling at $30 \mathrm{m} {\mathrm{s}}^{-1}$ throws a ball out over the bonnet. Relative to the car the initial velocity of the ball is $20 \mathrm{m} {\mathrm{s}}^{-1}$ at $60°$ to the horizontal. The angle of projection of the ball with respect to the horizontal road will be: